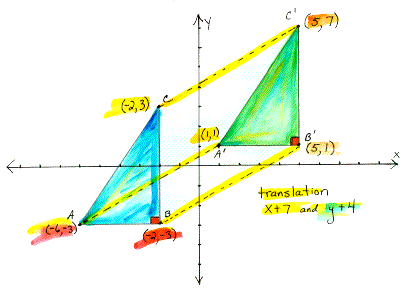Greek for Geo: 2012

Saturday, March 31, 2012

March 29: How do we find the surface area and lateral area of pyramids & cones? (AIM)

Aim: How do we find the surface area and lateral area of pyramids & cones?

So like last time's review, the surface area is the outside of a solid. So here we'll be working with solids like pyramids & cones. The similarity between these two solids is that they both have triangle(s).

Pyramids have triangles as the lateral area and only one face. This face can be any shape, from a circle (cone) to a heptagon (7 sides).

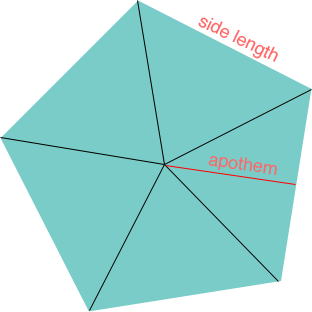
A pyramid in general is a solid that connects a polygon base to a point, called the apex.

For the surface area & lateral area this is the formula.

But remember this is only for a square pyramid. For any base use the formula of 1/2pl (perimeter x slant length) + B (area of the base).

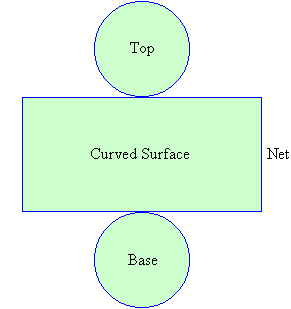
For a cone it's the same, but its for a circular base.

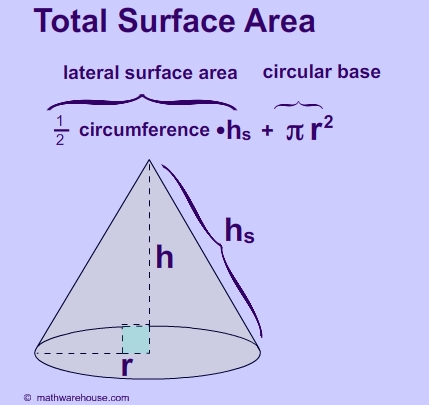
Well that's about it. So try this problem !

What is the total surface area of this cone, rounded to

the nearest tenth ?

Sources Cited:

March 28: How do we calculate the surface area of cylinders? (AIM)

Aim: How do we calculate the surface area of a cylinder?

To find the surface area of a any figure, you must have the right idea of what surface area is.

Surface area if one of the properties to a solid (which is 3-Dimensional). It covers the outside of the figure, unlike the volume (which is the inside).

When calculating the surface area of a cylinder, you should remember that it's always easier to break the figure down into smaller shapes. For example:

So this figure shows
how there are 2
circles & a rectangle
in a cylinder.

So for surface area you'll basically need the formula of a rectangle + 2 times the formula for a circle. Which gives you the SA formula.

So if that's how you find surface area, then how do you find lateral area? Well you basically just subtract the the bases.

So lateral area is
the rectangle of
the cylinder.

Now that this is somewhat down-packed, try this problem. Don't worry its not hard ?!

A cylinder has a radius of 8 inches and a height of 12 inches. What are the surface area of this cylinder?

SA = 320π
SA = 208π
SA = 480π

Sources Cited:

Sunday, March 25, 2012

March 17: How do we find the area of regular polygons? (AIM)

Aim: How do we find the area of regular polygons?

Regular polygons are polygons with all sides and all angles equal.

Some examples usually used as regular polygons are:

Pentagons - 5 (1/2 x a s)

There are 5 equal
triangles, with equal
side lengths.

Hexagons - 6 (1/2 x a s)

Heptagons - 7 (1/2 x a s)

But there's a shorter way to know this. Also remember that in any case n is number of sides, s is side length, and a is apothem [distance from center of polygon to the side].

This table shows that
the formula is 1/2 times
the # of sides, apothem,
and side length.

Also there's another rule for finding the area of a regular polygon. It's 1/2 x P x a. In this case P is the perimeter and a is the apothem.

Now try this problem:

What is n and the areas of this figure?

Sources Cited:

March 20: How do we find the area of a circle? (AIM)

Aim: How do we find the area of a circle?

A circle is known as a round plane figure whose boundary (the circumference) consists of points equidistant from a fixed center.

There are circles EVERYWHERE, seriously. Look at anything right now, & i will guarantee you can find a type of circle. For example is you have the iPhone, here's some circles on your phone (that you probably never let go of) :

These are just SOME circles
on the iPhone. Even the circles
i drew on there are CIRCLES man !

Now when finding the area of a circle, its just a little tricky. This is because there's 2 formulas for a circle. There's circumference (perimeter for circles) & area, but they're 2 different things. But they do have one thing the same, PI.

For circumference, you multiply the radius by 2 or multiply the diameter by Pi. Or go by the rule of either 2πr or πd. Either way they're both the same.

For area you'll square the radius and multiply it by Pi. Or the rule is πr².

The pink/purple shaded
part of the circle (inside),
is the area of a circle.

Here's an example of finding the area & circumference of a circle :

So now that you've got everything packed down on area & circumference ... try this problem out :

What is the area of the colored part this circle ?

Sources Cited:

Saturday, March 17, 2012

March 15: How do we find the area of parallelograms, kites, and trapezoids ? (AIM)

Aim: How do we find the area of parallelograms, kites, and trapezoids ?

The area of a shape is the amount of space inside the boundary of a flat (2-dimensional) object.

Here's a video link that can help you understand it more: http://www.brainpop.com/math/geometryandmeasurement/areaofpolygons/

On that link you can watch the video, try the activity, take a quiz on it, & etc. But I will still try to explain it here.

The area for a parallelogram is probably the easiest. Just because you only have to multiply length and height (l x w). But there is an easier way to look at it.

Now the area of a kite is very different. You have to multiply the 2 diagonals and then divide that by 2. ((d1 x d2) / 2)

The area for a trapezoid is kind of similar to the kite's, but instead of diagonals there are bases & a hieght. In this case you add the 2 bases and then multiply that by the half of the height.

So after all this wonderful information, try this problem here !

Sources Cited:

http://savannahsmathblog.blogspot.com/2010/04/area-and-volume.html
http://www.k6-geometric-shapes.com/kite-shape.html
http://www.algebra-class.com/area-formula.html
John Schnatterly AKA --> http://johnschnatterly.blogspot.com/

Tuesday, March 13, 2012

March 12: How do we calculate the area of rectangles and triangles? (AIM)

Aim: How do we calculate the area of rectangles and triangles?

Well for starters, the are of a rectangle is very different from the area of a triangle.

The area of a triangle has a formula like this:

Area would equal
1/2 times the base
and height.

The area of a rectangle has a formula like this:

The length is multiplied
by the width.

Sometimes in a question, you'll get a shape that looks kind of funny. But if you break it down, there might be a triangle or/and a rectangle. For example in this problem:

What would be the area
for this figure?

So you would break it down like this:

Let's break it down into two parts.
Part A is a rectangle,
& Part B is a triangle.

Part A is a square:

Area of A = w x h = (20m) × (20m) = 400m²

Part B is a triangle:

Area of B = ½(b × h) or (b × h)/2 = ½ × (20m × 14m) = 140m²

Now to find the area of that figure you saw earlier, you add the 2 areas:

Area = (Area of A) + (Area of B) = 400m² + 140m² = 540m²

Now you try it !

What is the area of this triangle ?

30

90

56

28

Sources Cited:

Saturday, March 10, 2012

March 5: How do we find the locus of points? (AIM)

Aim: How do we find the locus of points?

When finding the locus or the locus of points, you should go by this definition:

- the set of all points that satisfy a given condition.

The locus is usually graphed on a general graph of a given equation. but this is only sometimes, depending on the question (what is asked).

A single point:

When graphing the locus of points that are equidistant from a single point, it'll create a circle with the original point at it's center point. Also with the set of points in the line of the circle !

2 points:

When graphing the locus of points that are equidistant from 2 points , it'll be the perpendicular bisector of the line segment connecting the 2 points. This forms a line through the middle of the 2 points.

The 2 points which are P & Q
have a line that makes a perpendicular
bisector through the line that P & Q.

A line:

When graphing the locus of points that are equidistant from a line are 2 lines (that are on opposite sides), it'll be the same distance [equidistant] and parallel to the line. This leaves 2 parallel lines on opposite sides of the original line.

2 lines:

When graphing the locus of points that are equidistant from 2 parallel lines is another line (half-way between both lines), then each of the them will be parallel. So it'll be a line through the middle of the 2 lines.

These locus of points are equidistant
from the y-axis. They are on the
lines x=-1.5 and x=1.5.

2 intersecting lines:

When graphing the locus of points that are equidistant from 2 intersecting lines, it'll bisect the angles formed by the original lines. That means that the 2 intersecting lines are half-way between the 2 original lines.

Line 1 and Line 2 intersect at the origin.

Sources Cited:

Sunday, March 4, 2012

Aim: How do we solve logic problems using conditionals?

Aim: How do we solve logic problems using conditionals?

Well when solving logic problems using conditionals, you have to use any conditional right.

- a conditional is a most used statement in an argument of math.

There are four conditionals, they are :

Conditional( = Converse)
Inverse (same as Contrapositive)
Converse ( = Conditional)
Contrapositive (same as Inverse)

But there's also Biconditional, which is when both conditional & converse are TRUE.

When dealing with conditionals you have to identify the hypothesis and conclusion of a conditional.

Now Try it with this little segment !

1.		Which of the following statements is the converse of"If the moon is full, then the vampires are prowling."?
	Choose one: If the vampires are prowling, then the moon is full. If the moon is not full, then the vampires are prowling. If the vampires are not prowling, then the moon is not full. (correct answer: 1)

Sources Cited:

John Schnatterly AKA --> http://johnschnatterly.blogspot.com/

http://regentsprep.org/Regents/math/geometry/GP2/PracRC.htm

Aim: What is a mathematical statement ?

Aim: What is a mathematical statement ?

What is logic to you? What do you use Logic for ? Pretty much everything, right ?!

A mathematical statement is

- a statement that can be judged to be true or false.

This kind of statement(s) uses "and" when both statements put together are TRUE. When the statement(s) use "or" then the statements both could either TRUE or just one is TRUE.

This also connects to Conjunctions and their Functions.

A brief thought on Conjunctions.

But our main question from this is ....

Am I right OR what ???

Why don't we ask our conjunction conductor about the conjunctions and their functions !

So from this video we get that you should remember the phrase "FANBOYS", wanna know why? because:

These are the most
used words for
conjunctions.

Here's a little problem that can help when using conjunctions in a mathematical statement:

What word would be used to make the statement "That pencil is ____ her Junior !" TRUE?

a)Yet
b)Or
c)So
d)For

Sources Cited:

Aim: What is logic ?

Aim: What is logic?

Logic is thinking ! Its the tool to determine between whats true & whats false.

Logic is using proofs to prove that the truth is being told in a composition.

This shows how subjects go
from converse and inverse.

You can also use Conditionals to solve logic problems. But you have to make sure you use the right one. A conditional is :

- a most frequently used statement in an argument or in the study of math (ie.geometric proofs)

There are also different types of conditionals and more !

These are the four types of Conditionals.

To help explain this a little bit more, here's a brief statement on conditionals.

But for now, just try this little logic problem to get that noggin working !

How would you write a conditional statement from the hypothesis of "three and five are combined" and the conclusion of "it is equal to eight" ?

a) If it is equal to eight, then three and five are combined.
b) If three and five are not combined, then it is not equal to eight.
c)If three and five are combined, then it is equal to eight.

Sources Cited:

Sunday, February 19, 2012

Aim: How do we graph rotations?

Rotation is when an image is rotated from one point with the same distance. So here are some rules when it comes to rotating.

90 degrees: (x,y) → (-y,x)
180 degrees: (x,y) → (-y,-x)
270 degrees: (x,y) → (y,-x)

These rules are for counter-clockwise, except for 180 degrees. Now if a problem asks for clockwise, you should use the rule that is opposite. For example:

If it says "90 degrees clockwise", you could use the rule for 270 degrees counter-clockwise.
If it says "270 degrees clockwise", you could use the rule for 90 degrees counter-clockwise.

This globe/earth is going
360 degrees counter-clockwise.

This is because if you look at a clock and try what a question is asking, it'll make sense. Just because if your going 90 degrees clockwise, it'll be the same as going 270 counter-clockwise. And if your going 180 degrees, it'll be the same either counter-clockwise or clockwise.

Now try it yourself !

↓

Aim: How do we use the other definitions of transformations?

There are 4 main types of transformations, but there are many more. The main four are:

Rotation: The distance from the center to any point on the shape stays the same.

This ballerina is rotating
360 degrees consistantly.
She is rotating from her heel
on her left foot.

Translation: When an image moves by changing it's coordinates by equal distance, same direction, and that the figure stays the same size.

A students drawing of their
ΔABC being translated by T(7,4).

Reflection: when an image is reflected over a line to create a mirror image.

The woman in this picture is
being reflected by the
lake in front of her.

Dilation: when an image is enlarged or shrunk from its original being.

The image that Homer is
holding, is the image that
the screen keeps
dilating into.

This person's pupil is
dilated due to being horrified.

There are many more transformations out there ! But try this little problem:

Aim: What is Isometry?

The definition of Isometry:

- Basically the distance from an original figure (& it's points) and their transformed figure (& their points).

An example of how ΔABC's points are equally distanced
from ΔA'B'C' points. This is shown by translation.

From the types of Transformations only Reflection, Rotation, and Translation are isometries. Dilation on the other hand, is not a isometry.

This earth figure is isometry
b/c each half of the earth
is equal distance from
the other half.

The reason why dilation isn't a isometry is because the original image is either shrunk or enlarged.

Example of dilation, where
ΔABC is dilated by the
scale factor of 2.

Now you try it, with this question :

What isometry maps figure 1 to figure 3?

Choices:

A. reflection

B. translation

C. rotation

D. none of these

Greek for Geo

Saturday, March 31, 2012

March 29: How do we find the surface area and lateral area of pyramids & cones? (AIM)

March 28: How do we calculate the surface area of cylinders? (AIM)

Sunday, March 25, 2012

March 17: How do we find the area of regular polygons? (AIM)

March 20: How do we find the area of a circle? (AIM)

Saturday, March 17, 2012

March 15: How do we find the area of parallelograms, kites, and trapezoids ? (AIM)

Tuesday, March 13, 2012

March 12: How do we calculate the area of rectangles and triangles? (AIM)

Saturday, March 10, 2012

March 5: How do we find the locus of points? (AIM)

Sunday, March 4, 2012

Aim: How do we solve logic problems using conditionals?

Aim: What is a mathematical statement ?

Aim: What is logic ?

Sunday, February 19, 2012

Followers

About Me